Many scientists admit some equations are often fond of particular formulas not just for their function, but for their form.
While certain famous equations, such as Albert Einstein’s E = mc^2, hog most of the public glory, many less familiar formulas have their champions among scientists.
Here we show you the equations most voted as “the most beautiful in the world”:
General Relativity
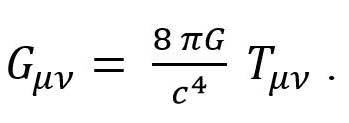
The equation above was formulated by Einstein as part of his groundbreaking general theory of relativity in 1915. The theory revolutionized how scientists understood gravity by describing the force as a warping of the fabric of space and time.
The Standard Model
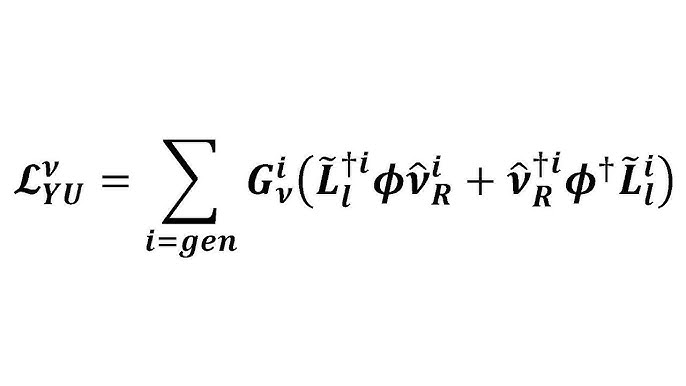
Another of physics’ reigning theories, the standard model describes the collection of fundamental particles currently thought to make up our universe.
The theory can be encapsulated in a main equation called the standard model Lagrangian (named after the 18th-century French mathematician and astronomer Joseph Louis Lagrange).
Calculus
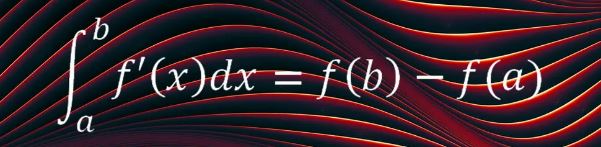
While the first two equations describe particular aspects of our universe, another favorite equation can be applied to all manner of situations. The fundamental theorem of calculus forms the backbone of the mathematical method known as calculus, and links its two main ideas, the concept of the integral and the concept of the derivative.
Pythagorean Theorem
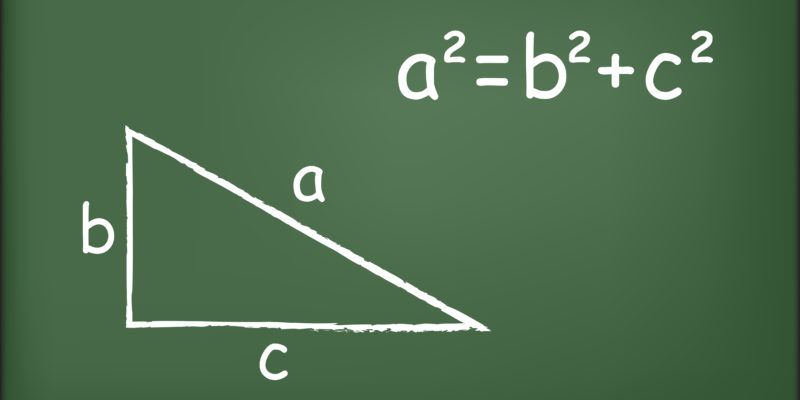
An “oldie but goodie” equation is the famous Pythagorean theorem, which every beginning geometry student learns.
This formula describes how, for any right-angled triangle, the square of the length of the hypotenuse, c, (the longest side of a right triangle) equals the sum of the squares of the lengths of the other two sides (a and b). Thus, a^2 + b^2 = c^2.
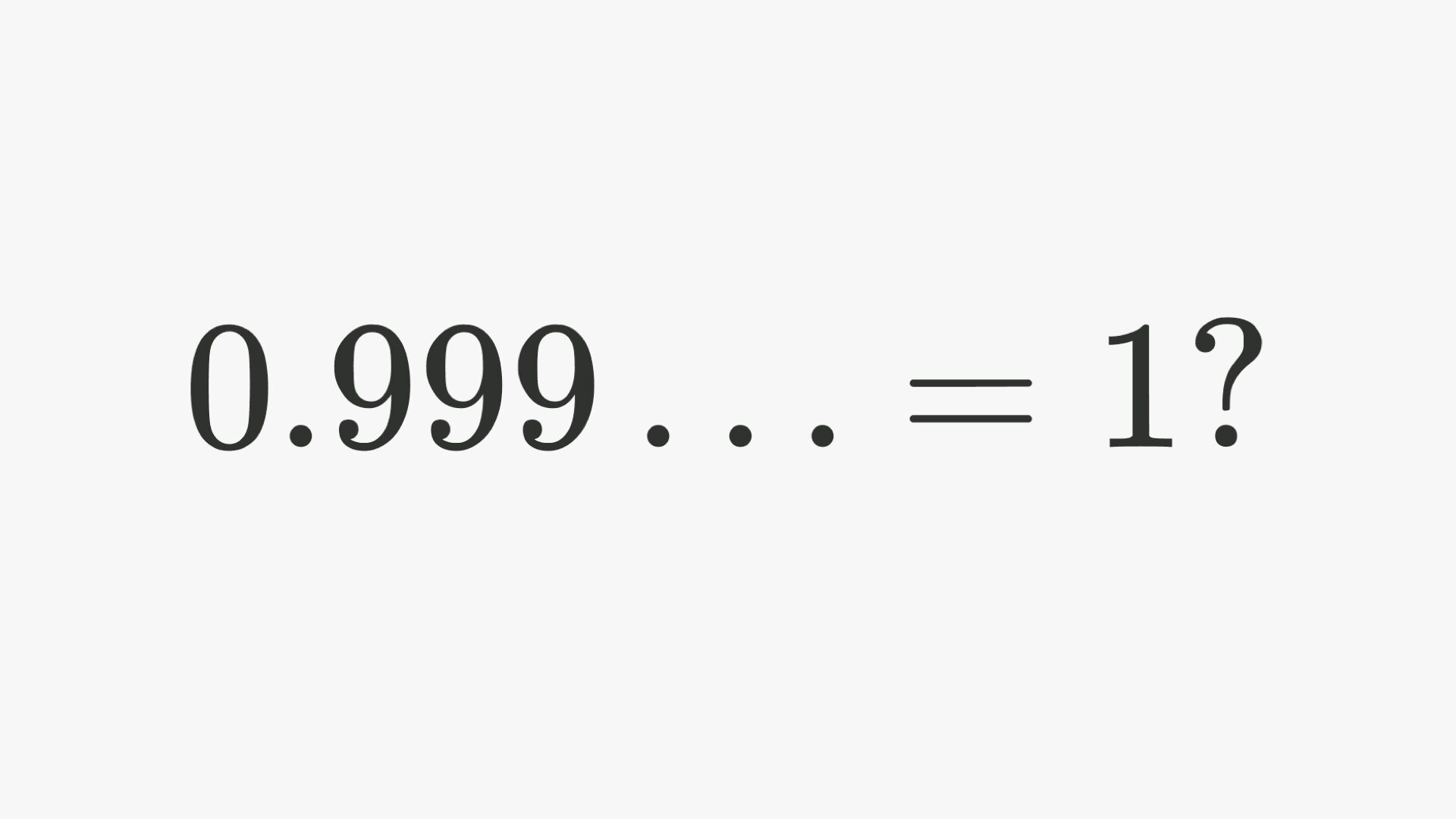
1 = 0.999999999…
This simple equation states that the quantity 0.999, followed by an infinite string of nines, is equivalent to one.
Special Relativity
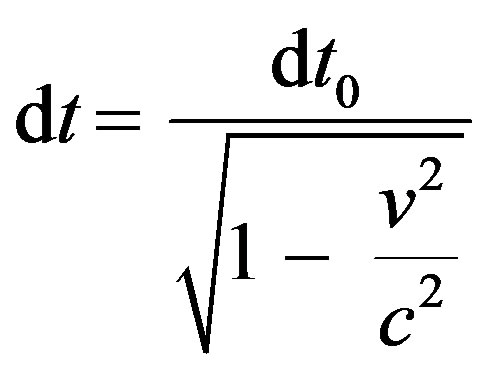
Einstein makes the list again with his formulas for special relativity, which describes how time and space aren’t absolute concepts, but rather are relative depending on the speed of the observer. The equation above shows how time dilates, or slows down, the faster a person is moving in any direction.
Euler’s Equation
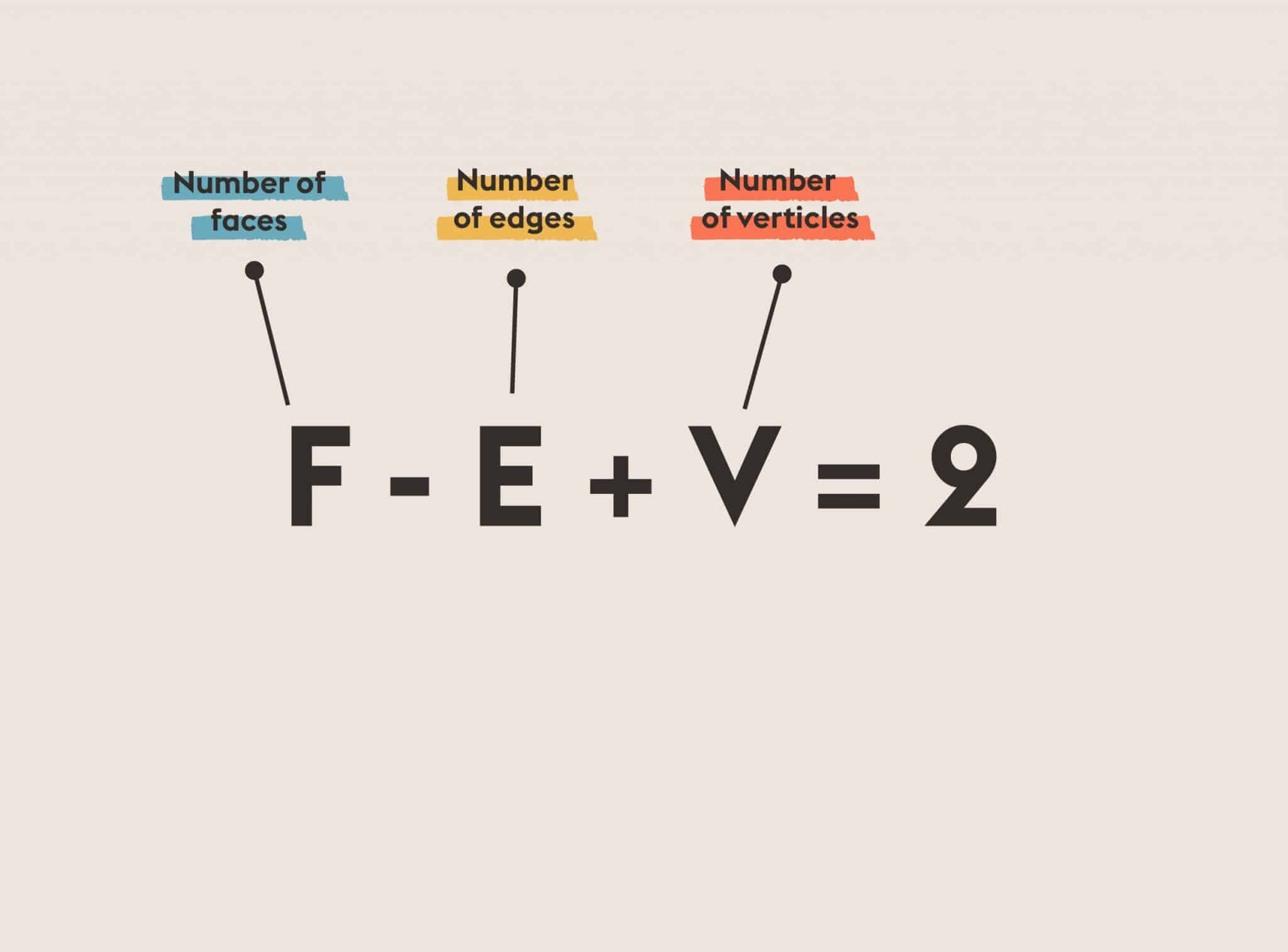
This simple formula encapsulates something pure about the nature of spheres:
If you cut the surface of a sphere up into faces, edges and vertices, and let F be the number of faces, E the number of edges and V the number of vertices, you will always get V – E + F = 2.
Euler-Lagrange Equations and Noether’s Theorem
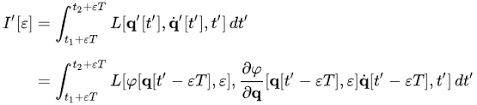
Here, L stands for the Lagrangian, which is a measure of energy in a physical system, such as springs, or levers or fundamental particles. “Solving this equation tells you how the system will evolve with time”.
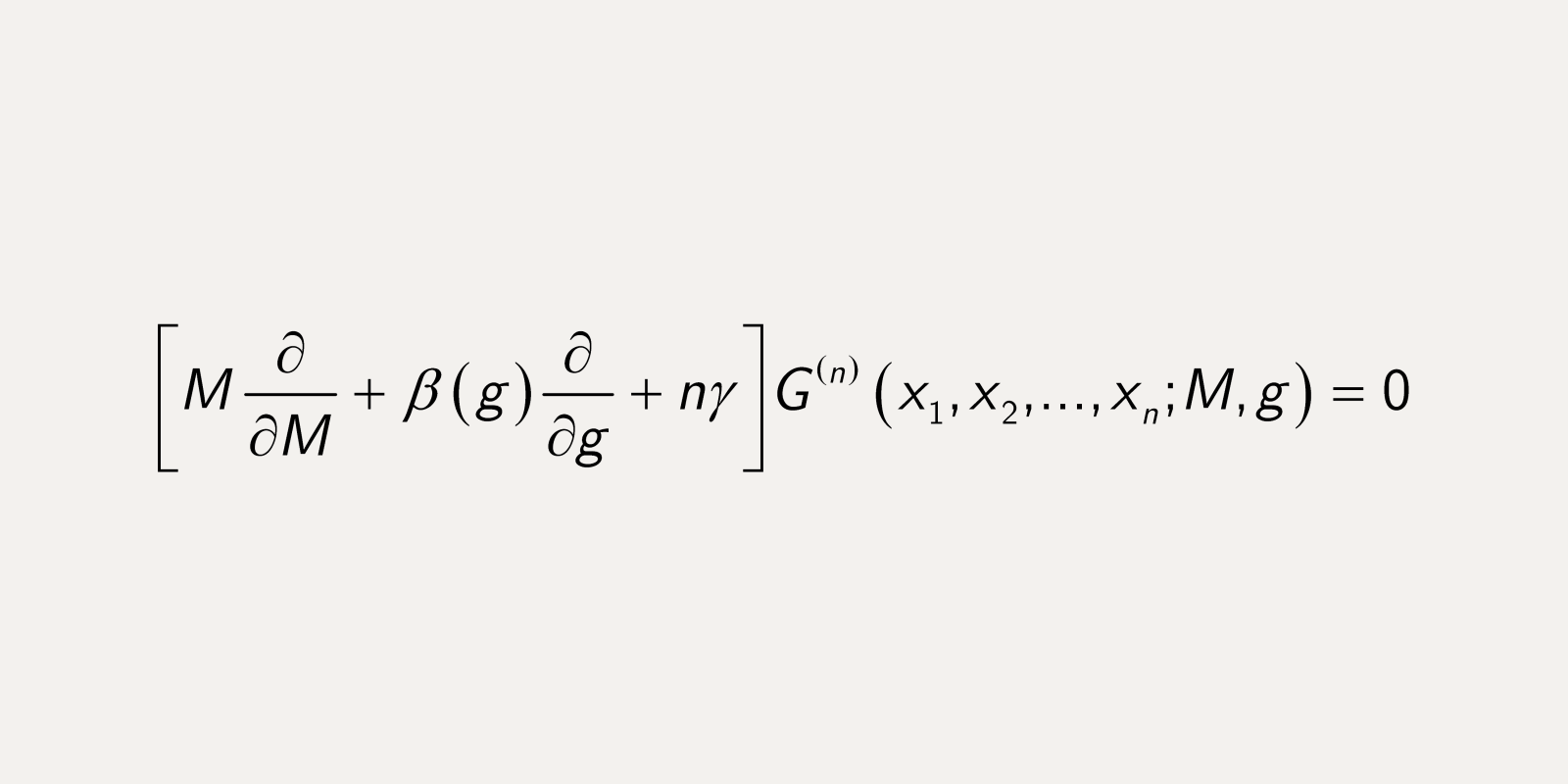
The Callan-Symanzik Equation
The equation has numerous applications, including allowing physicists to estimate the mass and size of the proton and neutron, which make up the nuclei of atoms.
The Minimal Surface Equation
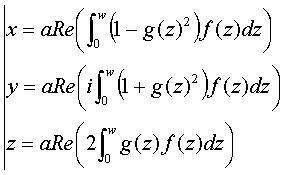
The minimal surface equation somehow encodes the beautiful soap films that form on wire boundaries when you dip them in soapy water. The fact that the equation is ‘nonlinear,’ involving powers and products of derivatives, is the coded mathematical hint for the surprising behavior of soap films. This is in contrast with more familiar linear partial differential equations, such as the heat equation, the wave equation, and the Schrödinger equation of quantum physics.
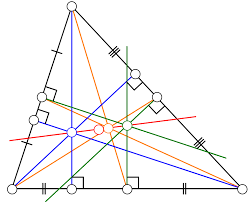
The Euler Line
It’s a line determined from any triangle that is not equilateral. It is a central line of the triangle, and it passes through several important points determined from the triangle, including the orthocenter, the circumcenter, the centroid, the Exeter point and the center of the nine-point circle of the triangle.
Fuente: LiveScience


 Clase Espejo México-Colombia: Un puente académico sobre el cambio climático #ESCAT #Uninter #MedioAmbiente
Clase Espejo México-Colombia: Un puente académico sobre el cambio climático #ESCAT #Uninter #MedioAmbiente